The Geometry of LOVE
Love squared by Love
rounding each edge,
geometrizing each ends
equalizing its alphabets,
circling its triangles.
Infinitesimally surrounding
transitory planes and lanes
within our pyramidal
silences,
giving new lines and
directions,
intersections of
re-constructions
compasses and conjunctures
within rebellious
Mathematics
Endless rounded prismatic
longings
leaving behind the theory of
angles
rising trigonometry of the
hearts
forgetting about scientific
breaths
inside seven circles
oscillating harmonies
Love squared by Love
converting the Word into ART
orbiting among infinite
numbers of points
till can be found just a
line between me and you
galactically entangled,
universally connected
with simplified distraction,
amplified seduction
sometimes equivalent,
sometimes equidistant
and the sum of the cosmic
Algebra in two hearts.
Love squared by Love
in perpendicular stars and
parallel moons
crossing the lines in
algorithmic dances
and waves of psychedelic
sensations,
kabbalistic stardust
hologram inceptions
rhythmic complexities and
elastic canvas.
Neither perimeters nor
cross-sections,
neither postulates nor heart
formula
when I am blue and you are
green,
answers not to be based on
x-y-z coordinates
or figure-ing out to be even
but ever-being presently
present!
Love squared by Love
applied symmetries and
Platonic shapes,
an amalgamation of binaries
and analogues
sometimes with no common
denominators,
no obtuse views but endless
Mandelbrotian spirals
where human is able to
accept a simple deviation.
Love squared by Love
embracing your concavity
into my convex world,
summing up the trigonometry
of our cosmic hearts
As LOVE tangles between two
dots…
Love!
Not a mundane Geometry!
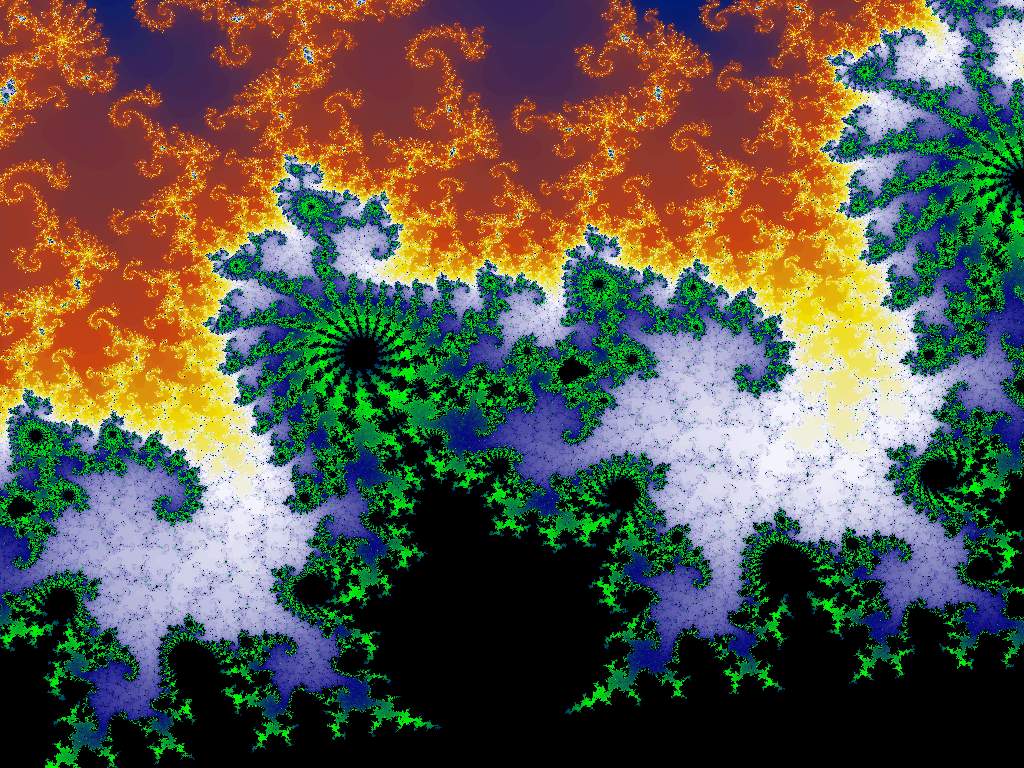Many-Pointed Many-Budded Buds -- Anton Feenstra
Benoit B. Mandelbrot added the middle initial to his name, not intending it to stand for a middle name but to recursively mean Benoit B. Mandelbrot, thereby including a fractal in his own name. He called himself a “fractalist” rather than a mathematician. His autobiography, “The Fractalist: Memoir of a Scientific Maverick,” was published in 2012, two years after his death. In “Science” in 1967 he published an article, “How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension,” in which he discussed self-similar curves. In 1974 he used his new insights to offer a solution of Olbers' paradox that a static, infinitely old universe with an infinite number of stars distributed in an infinitely large space would be bright rather than dark. [The paradox is named after the 19th-century amateur German astronomer Heinrich Wilhelm Olbers but had earlier been suggested by Thomas Digges, who had been the first to expound the Copernican system in English and also postulated an infinite universe with infinitely many stars, and had been systematically developed in the 1th century by Edmond Halley and Jean-Philippe Loys de Chéseaux; Edgar Allan Poe had even anticipated its first (1901) satisfactory resolution by William Thomson (Lord Kelvin), in his 1848 “Eureka: A Prose Poem”: “Were the succession of stars endless, then the background of the sky would present us a uniform luminosity, like that displayed by the Galaxy – since there could be absolutely no point, in all that background, at which would not exist a star. The only mode, therefore, in which, under such a state of affairs, we could comprehend the voids which our telescopes find in innumerable directions, would be by supposing the distance of the invisible background so immense that no ray from it has yet been able to reach us at all.”]
ReplyDeleteIn 1977 Mandelbrot introduced his ideas in “Fractals: Form, Chance and Dimension.” As an IBM fellow at the Thomas J. Watson Research Center in Yorktown Heights, New York, he had access to the company’s computers and was one of the first to use computer graphics to create and display fractal geometric images, leading to his discovery of the Mandelbrot set in 1979. He showed how visual complexity can be created from simple rules, showing that things typically considered to be "rough," a "mess," or "chaotic" (like clouds or mountains, fern leaves or galaxies, blood vessels or lungs) actually had a "degree of order." In 1982, he expanded and updated his ideas in “The Fractal Geometry of Nature.” Fractals are geometric shapes that are equally "rough" at all scales, never becoming simpler. Smaller and smaller copies of a pattern are successively nested inside each other, so that the same intricate shapes continue to appear. A rocky coastline looks just as jagged at one’s feet as from outer space. In an interview with science fiction writer Arthur C. Clarke, he modestly denied having any “feeling of invention. I never had the feeling that my imagination was rich enough to invent all those extraordinary things on discovering them. They were there, even though nobody had seen them before. It's marvelous, a very simple formula explains all these very complicated things. So the goal of science is starting with a mess, and explaining it with a simple formula, a kind of dream of science.” Clarke, however, effusively claimed "the Mandelbrot set is indeed one of the most astonishing discoveries in the entire history of mathematics. Who could have dreamed that such an incredibly simple equation could have generated images of literally infinite complexity?" He also noted the similarity between Mandelbrot’s name and the word “mandala” (Sanskrit for “circle”), the spiritual and ritual symbol that represents the universe. Fractals are discernable in many human pursuits such as music, painting, architecture, and stock market prices and have played an important role in the development of statistical physics, meteorology, hydrology, geomorphology, anatomy, taxonomy, neurology, linguistics, information technology, economics, geology, medicine, cosmology, engineering, chaos theory, econophysics, metallurgy, taxonomy and the social sciences.
ReplyDelete